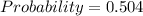Answer:
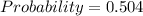
Explanation:
Given
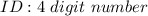
Required
Determine the probability that no two digit is repeated
First, we need to determine the total possible number of pins (with repetition)
Each of the 4 ID digit can be selected from any of the 10 digits (0 - 9).
i.e.
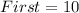

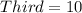
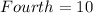

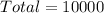
i.e.
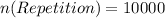
Next, we determine the total possible number of pins (without repetition)
Once a digit is selected from any of the 10 digits (0 - 9), it can no longer be repeated.
i.e.
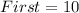
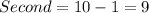
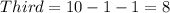

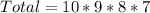
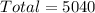
i.e.
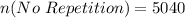
The probability is then calculated as: