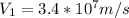Question

To initiate a nuclear reaction, an experimental nuclear physicist wants to shoot a proton into a 5.50-fm-diameter 12C nucleus. The proton must impact the nucleus with a kinetic energy of 2.30 MeV. Assume the nucleus remains at rest. With what speed must the proton be fired toward the target?

Answer 1

`V_1= 3.4*10^7m/s`

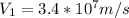
Step-by-step explanation:

From the question we are told that

Nucleus diameter
`d=5.50-fm`

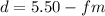
a 12C nucleus

Required kinetic energy
`K=2.30 MeV`

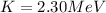
Generally initial speed of proton must be determined,applying the law of conservation of energy we have

`K_2 +U_2=K_1+U_1`

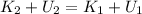
where

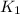`K_1` =initial kinetic energy

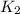`K_2` =final kinetic energy

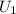`U_1` =initial electric potential

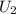`U_2` =final electric potential

mathematically

`U_2 = (Kq_pq_c)/(r_2)`

where

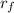`r_f`=distance b/w charges

`q_c`=nucleus charge
`=6(1.6*10^-^1^9C)`

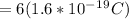
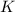`K`=constant

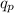`q_p`=proton charge

Generally kinetic energy is know as

`K=(1)/(2) mv^2`

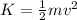
Therefore

`U_2 = (Kq_pq_c)/(r_2) + K_2=(1)/(2) mv_1^2 +U_1`

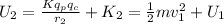
Generally equation for radius is
`d/2`

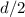
Mathematically solving for radius of nucleus

`R=((5.50)/(2)) ((1*10^-^1^5m)/(1fm))`

`R=2.75*10^-^1^5m`

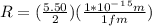
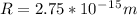
Generally we can easily solving mathematically substitute into v_1

`q_p`
`=6(1.6*10^-^1^9C)`

`K_1=9.0*10^9 N-m^2/C^2`

`U_1= 0`

`R=2.75*10^-^1^5m`

`K=2.30 MeV`

`m= 1.67*10^-^2^7kg`

`V_1= ((2)/(1.67*10^-^2^7kg))^1^/^2 (((9.0*10^9 N-m^2/C^2)*(6(1.6*10^-^1^9C)(1.6*10^-^1^9C))/(2.75*10^-^1^5m+2.30 MeV((1.6*10^-^1^3 J)/(1 MeV)) )`

`V_1= 3.4*10^7m/s`

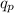
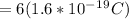
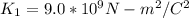
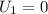
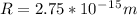
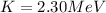
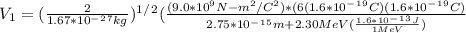
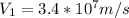
Therefore the proton must be fired out with a speed of
`V_1= 3.4*10^7m/s`