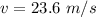Answer:
The value is
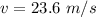
Step-by-step explanation:
From the question we are told that
The velocity is
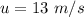
The length of the slope is
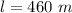
The height of the slope is
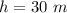
The mass of the cyclist and her bicycle is
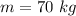
The drag force is
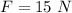
Generally from the law of energy conservation we have that
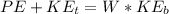
Here
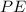 is the potential energy at the top of the slope which is mathematically represented as
is the potential energy at the top of the slope which is mathematically represented as
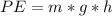
=>
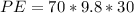
=>

and
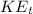 is the kinetic energy at the top of the slope which is mathematically represented as
is the kinetic energy at the top of the slope which is mathematically represented as

=>

=>
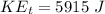
And W is the work done by the bicycle which is mathematically represented as

=>
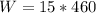
=>

and
 is the kinetic energy at the bottom of the slope which is mathematically represented as
is the kinetic energy at the bottom of the slope which is mathematically represented as
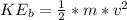
=>
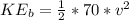
=>

So

=>