Answer:
a) k = 3.2 N / m
b) T = 0.27 s
some problem measuring the time of the oscillation.
Step-by-step explanation:
a) With the student's observations we can make a graph of elongation against applied weight, see attached, with this graph, Hooke's law must comply
F = -k x
where force is the applied weight
F = W = mg
substituting
W = k x
x = 1 / k W (1)
the linear regression of the graph gives
x = 31.2 w + 0.23
the slope of the graph is
m = 31.2 cm/N
if we relate this value with equation 1
1 / k = 131.2
k = 0.032 N / cm
Let's reduce to the SI system
k = 0.032 N / cm (100cm / 1m)
k = 3.2 N / m
This is the best value we can obtain from the spring constant with these experiential data
b) When the student oscillates the system, he has a simple harmonic motion whose angular velocity is
w =
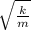
for the masses of m = 60 gr = 60 10-3 kg = 0.060 kg
w = √ (3.2 / 0.060)
w = 22.82 rad/s
angular velocity is related to frequency and period
w = 2π f = 2π / T
T = 2π / w
T = 2π / 22.82
T = 0.27 s
A great discrepancy is observed between the theoretical value and the experimental value, it is not common erorr so high it can be due to some problem measuring the time of the oscillation.