Answer:
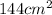
Explanation:
Step 1: Find the true length of each side
We know that the perimeter is 56cm and the ratio of AD:AB:DC:BC is 5:12:6:5. It is safe to assume that this ratio is the simplified version, which means that if we multiply each of these numbers by a scale factor of
 , then we get the true length of each side, so we would get
, then we get the true length of each side, so we would get
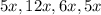 for the sides. We also know that when we add these numbers, we get the perimeter, which is 56. So, we can create the equation
for the sides. We also know that when we add these numbers, we get the perimeter, which is 56. So, we can create the equation
 . Then, solve the equation for x, which would be 2, and go back and multiply each number with 2 to get the following:
. Then, solve the equation for x, which would be 2, and go back and multiply each number with 2 to get the following:
AD = 5*2 = 10cm, AB = 12*2 = 24cm, DC = 6*2 = 12cm, BC = 5*2 = 10cm
Step 2: Calculate the Height
Now we know the lengths of each side, lets take a look at the formula for the area of a trapezium:
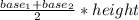 . So, the next step would be to calculate the height. We can draw the height by drawing a line straight down from the vertex D and another straight down from the vertex C. These lines are both the height, and will be the exact same length. We can calculate that the height is 8 because AB is 24 cm, but the length from the height at vertex D to the height at vertex C is 12 cm, so that means that the base of each triangle is 12/2 = 6 cm. Then we can use Pythagorean theorem to figure out that the height is 8cm.
. So, the next step would be to calculate the height. We can draw the height by drawing a line straight down from the vertex D and another straight down from the vertex C. These lines are both the height, and will be the exact same length. We can calculate that the height is 8 because AB is 24 cm, but the length from the height at vertex D to the height at vertex C is 12 cm, so that means that the base of each triangle is 12/2 = 6 cm. Then we can use Pythagorean theorem to figure out that the height is 8cm.
Step 3: Calculate the Area
Lastly, we can calculate the area. The formula is
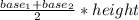 . In this case, the numbers would be
. In this case, the numbers would be
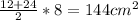
I know this is a lot, so let me know if you have any questions!