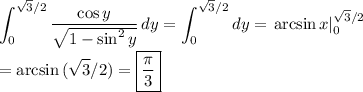Question

Solve the following question ​

Answer 1

9514 1404 393

Answer:

π/3

Explanation:

The given integral does not exist. We assume there is a typo in the upper limit, and that you want the integral whose upper limit is (√3)/2.

It is convenient to make the substitution ...

x = sin(y) . . . . so, y = arcsin(x)

dx = cos(y)·dy

Then the integral is ...

`\displaystyle\int_0^(√(3)/2){\frac{cos(y)}{\sqrt{1-\sin^2{y}}}}\,dy=\int_0^(√(3)/2){dy}=\left.\arcsin{x}\right|\limits_0^(√(3)/2)\\\\=\arcsin{(√(3)/2)}=\boxed{(\pi)/(3)}`