Question

2. Find the constant of variation k for the inverse variation. Then write an equation for the inverse variation.

y = 4.5 when x = 3
a. k = 13.5; xy = 13.5
b. k = 1.5; y = 1.5
x
c. k = 1.5; y = 1.5x
d. k = 13,5; 13.5y = x

Answer 1

Correct choice: a. k = 13.5; xy = 13.5

Explanation:

Inverse Proportion

Two variables x and y are in inverse proportion or variation if:

`\displaystyle y=(k)/(x)`

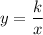
Where k is the constant of proportionality

Since we know that y=4.5 when x=3:

`\displaystyle 4.5=(k)/(3)`

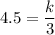
Solving for k:

`k = 4.5*3 = 13.5`

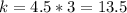
Thus the equation is:

`\displaystyle y=(13.5)/(x)`

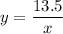
Or, equivalently:

`xy = 13.5`

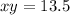
Correct choice: a. k = 13.5; xy = 13.5