Question

If you invest 11775.00 at a rate of 7.10 percent annum, compounded quarterly what will the total value of your investment be after 4 years

Answer 1

The total value of the investment will be $15,603.38 after 4 years

Explanation:

Compound Interest

It occurs when interest in the next period is earned on the principal sum plus previously accumulated interest.

We'll use the formula:

`{\displaystyle A=P\left(1+{\frac {r}{n}}\right)^(nt)}`

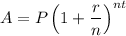
Where:

A = final amount

P = initial principal balance

r = interest rate

n = number of times interest applied per time period

t = number of time periods elapsed

The investment is P=$11,775 at a rate of r=7.10% = 0.071 compounded quarterly. Since there are 4 quarters in a year, n=4. The investment will last t=4 years, thus:

`{\displaystyle A=\$11,775\left(1+{\frac {0.071}{4}}\right)^(4*4)}`

`{\displaystyle A=\$11,775\left(1.01775\right)^(16)}`

`\boxed{A = \$15,603.38}`

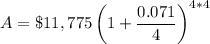
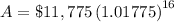
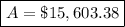
The total value of the investment will be $15,603.38 after 4 years