Question

Problem (11), 10 points 3 Math majors and 3 Computer Science majors will be presenting at a conference. Three students will be randomly selected to present on Monday, and the remaining three stu- dents will present on Tuesday.

(a, 3 points) What is the probability that two Computer Science majors and a math major will present on Tuesday? You do not need to simplify your answer.
(b, 5 points) Suppose we know at least one Math major has been selected to present on Monday. What is the probability that two Computer Science majors and a math major will present on Tuesday, given this information?
(c, 2 points) Based on your answer for b, are the events ‘at least one math major is selected to present on Monday' and 'two computer science majors and a math major present on Tuesday' independent?

Answer 1

a}
`P_1= 0.3`

b)
`P_2 =0.45`

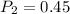
c) NOT INDEPENDENT

Explanation:

From the question we are told that

Sample space

Math majors are 3

computer science majors are 3

Generally the sample space is

Mathematically the sample space required on Monday is given as
`S_m= 3c_1 * 3C_2`

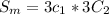
where
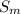`S_m` =sample space on Monday

Therefore,

`P_1` =
`(3C_1 *3C_2)/(6C_3)`

`P_1=(3*3)/(20)`

`P_1=(6)/(20)`

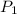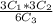
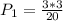
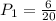
b)

Generally the the equation is given as

`P_2 =P((x \cap y)/(y))`

where

x is selecting two math major and 1 computer science major on Monday

y is at leas one math major selected

`P_2 =(^3C_1*^3C_2)/(^3C_1*^3C_2 + ^3C_2*^3C_1 +^3C_3*^3C_0)`

`P_2 =(3*3)/(3*3 + 3*3 +^1*1)`

c)

Generally in representing independent events

`P_3=p(x \cap y)`

=>
`p(x) * p(y)`

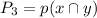
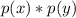
Mathematically

`n(x)= ^3C_1 *^3C_2`

`n(y)={^3C_1*^3C_2 + ^3C_2*^3C_1 +^3C_3*^3C_0}`

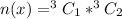
therefore

`n(x \cap y) =^3C_1 *^3C_2`

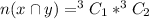
This does not satisfy the two equations stated above therefore NOT INDEPENDENT