Question

Need an answer showing work thax!

Answer 1

Option (a)
`\sum_(k=1)^(\infty) (3)/(k(k+1))`

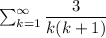
Explanation:

The given expression is :

`\frac{3}{1{\cdot} 2}+\frac{3}{2{\cdot} 3}+\frac{3}{3{\cdot} 4}+\frac{3}{4{\cdot} 5}+....`

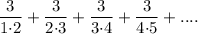
We need to rewrite the sum using sigma notation.

The numerator is 3 in all terms.

At denominator, in first term (1)(2), in second term (2)(3) and so on.

A general term for the denominator is k(k+1).

Sigma notation is :

`\sum_(k=1)^(\infty) (3)/(k(k+1))`

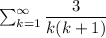
Hence, the correct option is (A).