Question

Triangle ABC is reflected across the y-axis to form the triangle A'B'C', then dilated

with a factor of 1/3 to form triangle A"B"C". Which of the following statements is
true for AABC and AA"B"C'?
O A) AABC and AAB"C" are similar triangles.
B)
There's not enough information to identify whether AABC and AA"B"C" are
congruent or similar.
C) AABC and AA"B"C"are congruent triangles.
D AABC and AA"B"C" are neither similar nor congruent.

Answer 1

Given:

Triangle ABC is reflected across the y-axis to form the triangle A'B'C'.

Then dilated with a factor of 1/3 to form triangle A"B"C".

To find:

The correct statement for triangles ABC and A"B"C".

Solution:

We know that reflection is a rigid transformation, it means size and shape of the figure remains same after reflection.

So, figures are always congruent to their images after reflection.

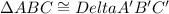`\Delta ABC\cong Delta A'B'C'` ...(i)

Dilation is not a rigid transformation. Here, shape remains same nut the size is different.

So, figures are always similar to their images after dilation.

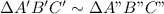`\Delta A'B'C'\sim \Delta A` ...(ii)

Using (i) and (ii), we get

`\Delta ABC\sim \Delta A`

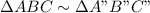
ΔABC and ΔAB"C" are similar triangles.

Therefore, the correct option is A.