Question

A wheel decelerates from 13.5 rad s−1 to 6.0 rad s−1 in 7 s. Calculate the angular displacement​

Answer 1

Angular displacement=68.25 rad

Step-by-step explanation:

Circular Motion

If the angular speed varies from ωo to ωf in a time t, then the angular acceleration is given by:

`\displaystyle \alpha=(\omega_f-\omega_o)/(t)`

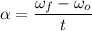
The angular displacement is given by:

`\displaystyle \theta=\omega_o.t+(\alpha.t^2)/(2)`

The wheel decelerates from ωo=13.5 rad/s to ωf=6 rad/s in t=7 s, thus:

`\displaystyle \alpha=(6-13.5)/(7)`

`\displaystyle \alpha=(-7.5)/(7)`

`\displaystyle \alpha=-1.071 \ rad/s^2`

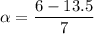
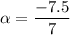
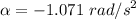
Thus, the angular displacement is:

`\displaystyle \theta=13.5*7+(-1.071*7^2)/(2)`

`\displaystyle \theta=94.5-26.25`

`\boxed{\displaystyle \theta=68.25\ rad}`

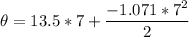
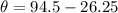
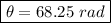
Angular displacement=68.25 rad