Question

2) A skier stands at rest and begins to ski downhill with an acceleration of 3.0 m/s² {downhill). What is

her displacement after 15.0 seconds?

Answer 1

337.5m

Step-by-step explanation:

Kinematics

Under constant acceleration, the kinematic equation holds:

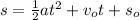`s=(1)/(2)at^2+v_ot+s_o`, where "s" is the position at time "t", "a" is the constant acceleration, "
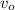`v_o`" is the initial velocity, and
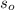`s_o` is the initial position.

Defining Displacement

Displacement is the difference in positions:
`s-s_o` or
`\Delta s`

`s=(1)/(2)at^2+v_ot+s_o`

`s-s_o=(1)/(2)at^2+v_ot`

`\Delta s=(1)/(2)at^2+v_ot`

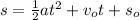
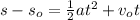
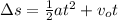
Using known information

Given that the initial velocity is zero ("skier stands at rest"), and zero times anything is zero, and zero plus anything remains unchanged, the equation simplifies further to the following:

`\Delta s=(1)/(2)at^2+v_ot`

`\Delta s=(1)/(2)at^2+(0)*t`

`\Delta s=(1)/(2)at^2+0`

`\Delta s=(1)/(2)at^2`

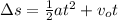
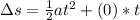
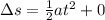
So, to find the displacement after 15 seconds, with a constant acceleration of 3.0 m/s², substitute the known values, and simplify:

`\Delta s=(1)/(2)at^2`

`\Delta s=(1)/(2)(3.0[(m)/(s^2)])(15.0[s])^2`

`\Delta s=337.5[m]`