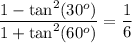Answer:
1/6
Explanation:
As usual in trigonometry, there are so many relationships between the trigonometric functions, there is bound to be more than one method to arrive at the answer.
Knowing values for the sine and cosine function for certain angles on the unit circle proves to be quite helpful. In general, I recommend 0°, 30°, 45°, 60°, and 90° at a minimum.
Recall the following 6 things:
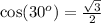
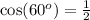


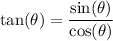
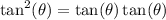
Knowing these 6 things (4 things from the unit circle, a way to write the tangent function in terms of sine and cosine, and the definition of a squared trig function), we can simplify the given expression down to a single number:
The definition of the tangent squared function is that the output of the tangent function is squared...
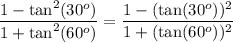
Using fact 5, and turning the tangent function into sines and cosines...
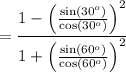
Using facts 1-4, and substituting known values for sines and cosines...
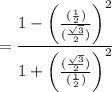
Division is multiplication by the reciprocal...
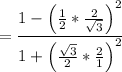
Reducing/simplifying common factors of 2...
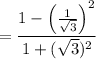
Squaring a square root...
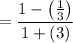
Finding a common denominator...
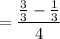
Definition of subtracting fractions...

Arithmetic...
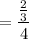
Division is multiplication by a reciprocal...
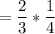
Simplification and reducing the fraction...
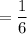
So,