Answer:
The amount of money after 12 years is $19581.99 to the nearest cents
Explanation:
The formula of the compound continuously interest is V = P
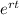 , where
, where
- V is the value of the account in t years
- P is the principal initially invested
- e is the base of a natural logarithm
- r is the rate of interest in decimal
∵ A person places $7320 in an investment account
∴ P = 7320
∵ The account earning an annual rate of 8.2%, compounded continuously
∴ r = 8.2% ⇒ divide it by 100 to change it to decimal
∴ r = 8.2 ÷ 100 = 0.082
∵ The time is 12 years
∴ t = 12
→ Substitute these values in the formula above to find V
∵ V = 7320

∴ V = 19581.99121 dollars
→ Round it to the nearest cents ⇒ 2 d.p
∴ V = 19581.99 dollars
∴ The amount of money after 12 years is $19581.99 to the nearest cents.