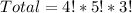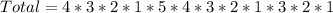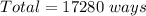Question

In how many ways can 5 different novels, 4 different mathematics books, and 1 biology book be arranged on a bookshelf if

Answer 1

Question Continuation:

if (a) the books can be arranged in any order?

(b) the mathematics books must be together and the novels must be together?

Answer:

(a) 3628800 ways

(b) 17280 ways

Explanation:

Given

`Novels = 5`

`Mathematics = 4`

`Biology = 1`

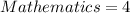
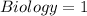
Solving (a): In any order

This implies that there is no restriction and the novels, mathematics and biology book are just seen as books.

Total number of books is:

`N = Mathematics + Novel + Biology`

`N = 4+5+1`

`N = 10`

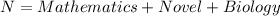
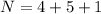
The number of arrangement is:

`10! = 10 * 9 * 8 * 7 * 6 * 5 * 4 * 3 * 2 * 1`

`10! = 3628800\ ways`

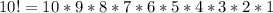
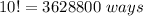
(b):

If the mathematics books are together, there are 4! different arrangements

If the novels are together, there are 5! different arrangements

Now, there are 3 bundles.

• The mathematics books as 1
• The novels as 1
• The biology book

This can be arranged in 3! ways.

Total number of arrangement is:

`Total = 4! * 5! * 3!`

`Total = 4*3*2*1 * 5*4*3*2*1 * 3*2*1`

`Total = 17280\ ways`