Answer:
a. V=44.9mL
Step-by-step explanation:
For a gas undergoing all of these changes, it will be important to combine Boyle's Law and Charles' Law to form the following equation (if it isn't already known):
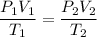
Where the Temperatures must be measured in Kelvin.
Recall that to convert Celsius to Kelvin, one must add 273 or use the equation
 .
.
Thus,
![T_1=(18.1+273)[K]=291.1[K]](https://img.qammunity.org/2023/formulas/chemistry/high-school/jko8mmwhaxps8ppcbvx6dfp34e75q3uo4l.png) and
and
![T_2=(12.5+273)[K]=285.5[K]](https://img.qammunity.org/2023/formulas/chemistry/high-school/8fd1eprtfg16ubr72lht9nz91j41wgt5ki.png)
To solve for the requested quantity, note that all of the other units match between beginning and end, so we substitute and solve:
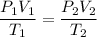
![((2.61[atm])V_1)/((291.1[K]))=((1.92[atm])(59.9[mL]))/((285.5[K]))](https://img.qammunity.org/2023/formulas/chemistry/high-school/5ikh0gmzou2td1vd2lepl37mc9ee4egwbb.png)
![\frac{(2.61[atm] \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!{-----})\bold{V_1}}{291.1[K] \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!{-----}}*\frac{291.1[K] \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!{-----}}{2.61[atm]\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!{-----}}=\frac{(1.92[atm]\!\!\!\!\!\!\!\!\!\!\!{--})(59.9[mL])}{285.5[K\!\!\!\!\!{-}]}*\frac{291.1[K\!\!\!\!\!{-}]}{2.61[atm]\!\!\!\!\!\!\!\!\!\!\!{--}}](https://img.qammunity.org/2023/formulas/chemistry/high-school/zidprsw5db7rq6ewk69ij1z6pcax8o1mwb.png)
![V_1=44.928677576[mL]](https://img.qammunity.org/2023/formulas/chemistry/high-school/ynyfo4b1zhjah4gr3lq0z99b0rpuaib727.png)
Accounting for significant digits,
![V_1=44.9[mL]](https://img.qammunity.org/2023/formulas/chemistry/high-school/qo6egft7rwfoyo1v1c091dpdufuddjz1i6.png)