Question

Movies Tonight is a typical video and DVD movie rental outlet for home-viewing customers. During the weeknight evenings, customers arrive at Movies Tonight with an arrival rate of 1.25 customers per minute. The checkout clerk has a service rate of 2 customers per minute. Assume Poisson arrivals and exponential service times. a. What is the probability that no customers are in the system

Answer 1

the probability having no customers are in the system is 0.375

Explanation:

The computation of the probability having no customers are in the system is as follows;

Given that the arrival rate is 1.25 customers per minute

The Service rate is 2 customers per minute

Based on the above information, the probability is

`=P_0\\\\=(1-(\lambda )/(\mu ))\\\\=(1-(1.25)/(2))\\\\=1-0.625\\\\= 0.375`

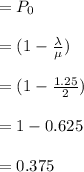
Hence, the probability having no customers are in the system is 0.375