Explanation:
Hey there!
The given equation of line PQ is :
y = -3x -12.
Comparing the equation with y = mx + c. We get;Slope (m1)= -3
We know the condition of parallel lines is slope of 1st line = slope of 2nd line. (i.e m1=m2).
Therefore, slope of PQ= slope of ST = -3.
Now, The point through which ST passes is (3,-1) and we found its slope as -3.
So, let's use one point formula.
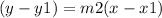
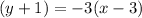
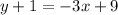
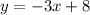
Comparing the equation with y = mx+c. We get y-intercept is "8".
Therefore, y-intercept is "8".