Answer:
The function which represents this sequence will be:
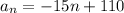
Hence, option (A) is true.
Explanation:
Given the sequence
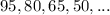
An arithmetic sequence has a constant difference 'd' and is defined by
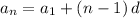
computing the differences of all the adjacent terms
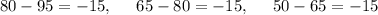
As the difference is the same, so
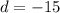
as

Thus, substituting
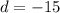 ,
,
 in the nth term of an arithmetic sequence
in the nth term of an arithmetic sequence
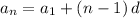
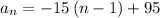
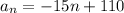
Therefore, the function which represents this sequence will be:
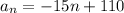
Hence, option (A) is true.