Question

6. What happens to the gravitational force between two objects if

b. The masses of both are doubled?
c. The distance between them is doubled?
d. The distance between them is cut in half?
please i need help

Answer 1

Step-by-step explanation:

The formula is

`F=(G*m_1*m_2)/(r^2)`

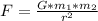
so anything making m larger increases the force

anything that decreases r makes F smaller.

B

So if you double each of the masses, the force becomes 4 times as large.

`F = (G*2m_1*2m_2)/(r^2)\\F = \frac{{4Gm_1*m_2}}{r^2}`

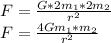
C

If the distance r is doubled, the force becomes 1/4 as large. That's because

r becomes 2r and when 2r is squared it becomes 4r^2. But that is in the denominator, so you are dividing by 4.

D

This is the hard one. You really have to understand fractions to know what is happening. it might be easier if we use decimals. Each r become 1/2 r or 0.5r

When you square that, you get 0.25 r^2

Just try it with something simple. Let r = 1 Now suppose that Gm1m2 = 20

20/0.25 = 80

When you 1/2 the distance the force is going to be 4 times as large.