Question

Bx = -1.33 m and By = 2.81 m.
Find the direction of the vector

Answer 1

The direction of the vector is 115.33°

Step-by-step explanation:

Polar Components of a Vector

One vector can be expressed in several forms. One of them is the polar form (r,θ) where r is the magnitude of the vector and θ is the angle formed by the vector and the positive x-axis direction.

If the rectangular components (x,y) of the vector are given, we can calculate the polar components as follows:

`r=√(x^2+y^2)`

`\displaystyle \tan\theta=(y)/(x)`

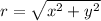
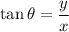
We are given the rectangular components of the vector B:

Bx= -1.33 m

By= 2.81 m

Note the vector lies on the second quadrant because the x-component is negative and the y-component is positive.

The direction of B is calculated below:

`\displaystyle \tan\theta=(2.81)/(-1.33)`

`\displaystyle \tan\theta= -2.1128`

`\displaystyle \theta= \arctan -2.1128`

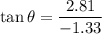
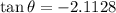
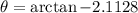
The scientific calculator gives:

`\displaystyle \theta= -64.67^\circ`

We need to add 180° to give the correct angle in the second quadrant:

`\theta= -64.67^\circ+180^\circ=115.33^\circ`

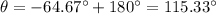
The direction of the vector is 115.33°