Question

100cm^3 of hydrogen is mixed with 25cm^3 of oxygen at a temperature of 150*C. The gases react as shown in the equation
`2H_(2) + O_2} -----\ \textgreater \ 2H_(2)O\\`

(All are gases)
What is the total volume of gas present at the end of the reaction?
50cm3
100cm3
125cm3
150cm3

explanation?
thx in advance

Answer 1

The total volume of gas present at the end of the reaction : 100 cm³

Further explanation

Given

100cm³ of hydrogen is mixed with 25cm³ of oxygen

Reaction

2H₂(g) + O₂(g) ===> 2H₂O(l)

Required

The total volume of gas

Solution

From Avogadro's hypothesis, at the same temperature and pressure, the ratio of gas volume will be equal to the ratio of gas moles

Find limiting reactant

mol ratio H₂(g) : O₂(g) from equation : 2 : 1, so volume ratio = 2 : 1

`\tt H_2/ O_2=(100)/(2)/ (25)/(1)=50/ 25`

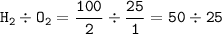
O₂ as a limiting reactant(smaller ratio), so the remaining H₂(as excess reactant) = 100 cm³ - 50 cm³(reacting hydrogen) =50 cm³

H₂O formed from O₂ = 2 x volume O₂ = 2 x 25 = 50 cm³

2H₂(g) + O₂(g) ===> 2H₂O(l)

100 25 0

50 25 50

50 0 50

Total volume gas present : volume H₂ + volume H₂O = 50 + 50 = 100 cm³