Question

Show that the de Broglie wave length of an electron in the ground state of Hydrogen atom corresponds to the circumference of the circle having the Bohr radius as its radius.

Answer 1

2
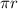`\pi r\\` = nλ

Step-by-step explanation:

Without mincing words let's dive straight into the solution to the above question. From the question above, we are given the need to start by using the de Broglie wavelength, this can be represented mathematically by the equation below:

h/ mv = λ. --------------------------------------------------------------------------------------------[1]. Where λ is the de Broglie wave length.

Starting by writing the mathematical representation for the angular momentum as given below:

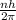`(nh)/(2\pi )` = mvr [ this is the mathematical representation for the angular momentum].

Thus, 2
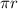`\pi r\\` = n[h/mv] ----------------------------------------------------------------------------[2].

Recall from equation [1] that h/ mv = λ. Therefore,

2
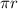`\pi r\\` = nλ. --------------------------------------------------------------------------------[3].

The equation above, that is equation [3] has proved that de Broglie wave length of an electron in the ground state of Hydrogen atom corresponds to the circumference of the circle having the Bohr radius as its radius.