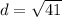Answer:
Point D:
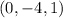
d = √41
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Pre-Calculus
- Midpoint Formula [3D]:
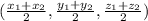
- Distance Formula [3D]:

Explanation:
Step 1: Define
Point A(1, 2, -1)
Point B(-3, -6, 2)
Point C(3, -2, 0)
Step 2: Find Point D
Simply plug in your coordinates B and C into the midpoint formula to find midpoint
- Substitute [MF]:
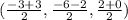
- Add/Subtract:
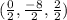
- Divide:
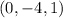
Step 3: Find distance d
Simply plug in the 2 coordinates A and D into the distance formula to find distance d
- Substitute [DF]:
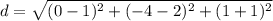
- Subtract/Add:

- Exponents:
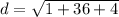
- Add: