Note: It seems, you missed to add the correct option. But, I am solving the question to clear your concept anways.
Answer:
The coordinates of H are:
Explanation:
Given the points
Since Point G and point H are the same distance from point F, therefore the point F is the mid-point of G and H
using the mid-point formula

Since the point F is the mid-point of G and H, so
m = (3, 2)
as
Thus, substituting m = (3, 2), (x₁, y₁) = (4, 4) to determine coordinates of the point H (x₂, y₂)

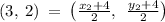
so
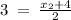

subtract 4 from both sides
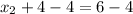
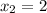
and
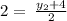
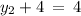
subtract 4 from both sides
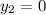
Thus, the coordinates of H are:
Note: It seems, you missed to add the correct option.