Answer:
d₂ = 6.18 mm
Step-by-step explanation:
We can work on this interesting exercise using the concepts of energy conservation
alpha (1) particle, with have a charge q = 2e
Starting point
Em₀ = U = q V₁
final point
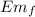 = ½ m v²
= ½ m v²
Em₀ = Em_{f}
2eV₁ = ½ m₁ v²
v² =
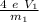
proton (2) particle, with have a charge q = e
Starting point
Em₀ = qV₂
Final point
Em_{f} = ½ m₂ v²
Em₀ = Em_{f}
eV₂ = ½ m₂ v²
v² =
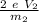
in the exercise, it should be noted that the two particles have the same velocity when reaching the plate, therefore let us solve the velocity in each equation and equal
\frac{4 \ e \ V_{1}}{m_{1} }= \frac{2 \ e \ V_{2} }{m_{2} }
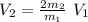
the alpha particle is composed of two protons and two neutrons, therefore in first approximation
m₁ = 4 m₂
subtitute
V₂ =
 V₁
V₁
let's calculate
V₂ =
 600
600
V₂ = 300 V
To find the distance we use the relationship between the electric field and the potential difference
V = -d E
The electric field between the plates is constant, so
E = - V / d = - V₂ / d₂
d₂ =
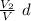
let's calculate
d₂ =
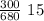
d₂ = 6.18 mm