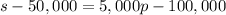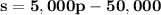Answer:

Explanation:
Linear Model
The supply of cartons of oil in January 2021 is predicted to reach 50,000 cartons for Tawfiq Trading Company with the carton selling for $20.
If we call s the supply of cartons and p the price, the ordered pair (p,s) for this condition is (20 ; 50,000).
It's predicted that the supply will fall by 20,000 if the price falls by 20%. The new supply will be 50,000-20,000=30,000 and the new price will be 0.8*$20=$16. This gives us the second point (16 ; 30,000)
The equation of a line passing through points (p1,s1) and (p2,s2) can be found as follows:
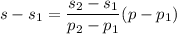
Substituting the given points:

Calculating:
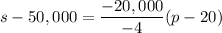
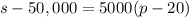
Operating and simplifying: