Understanding:
The sum of a geometric series is,
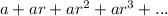 , with a being the start point and r is the common ratio. We can also use the following formula to make life easier
, with a being the start point and r is the common ratio. We can also use the following formula to make life easier
 , a is your start point, r is the common ratio, and n is the number of terms, which in our case is S7.
, a is your start point, r is the common ratio, and n is the number of terms, which in our case is S7.
Solution:
Our start point is 1,
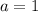 ,
,
The common ratio is 5,
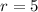 ,
,
And finally, the number of terms is 7,
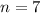 .
.
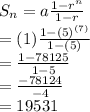
The answer is [A] 19531.