Answer:
Step-by-step explanation:
Half-life problems are modeled as exponential equations. The half-life formula is
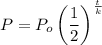 where
where
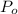 is the initial amount,
is the initial amount,
 is the length of the half-life,
is the length of the half-life,
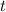 is the amount of time that has elapsed since the initial measurement was taken, and
is the amount of time that has elapsed since the initial measurement was taken, and
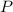 is the amount that remains at time
is the amount that remains at time
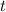 .
.
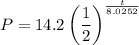
Deriving the half-life formula
If one forgets the half-life formula, one can derive an equivalent equation by recalling the basic an exponential equation,
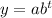 , where
, where
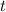 is still the amount of time, and
is still the amount of time, and
 is the amount remaining at time
is the amount remaining at time
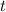 . The constants a and b can be solved for as follows:
. The constants a and b can be solved for as follows:
Knowing that amount initially is 14.2g, we let this be time zero:
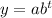
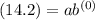
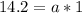
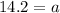
So,
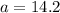 , which represents out initial amount of the substance, and our equation becomes:
, which represents out initial amount of the substance, and our equation becomes:

Knowing that the "half-life" is 8.0252 days (note that the unit here is "days", so times for all future uses of this equation must be in "days"), we know that the amount remaining after that time will be one-half of what we started with:
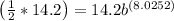
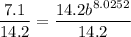

![\sqrt[8.0252]{(1)/(2)}=\sqrt[8.0252]{b^(8.0252)}](https://img.qammunity.org/qa-images/2023/formulas/physics/college/c1j9dmms0dppu20ygfdk.png)
![\sqrt[8.0252]{(1)/(2)}=b](https://img.qammunity.org/qa-images/2023/formulas/physics/college/36bmqgosxio9s56dq87m.png)
Recalling exponent properties, one could find that
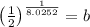 , which will give the equation identical to the half-life formula. However, recalling this trivia about exponent properties is not necessary to solve this problem. One can just evaluate the radical in a calculator:
, which will give the equation identical to the half-life formula. However, recalling this trivia about exponent properties is not necessary to solve this problem. One can just evaluate the radical in a calculator:
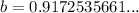
Using this decimal approximation has advantages (don't have to remember the half-life formula & don't have to remember as many exponent properties), but one minor disadvantage (need to keep more decimal places to reduce rounding error).
So, our general equation derived from the basic exponential function is:
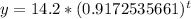 or
or
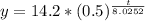 where y represents the amount remaining at time t.
where y represents the amount remaining at time t.
Solving for the amount remaining
With the equation set up, substitute the amount of time it takes to cross the Pacific to solve for the amount remaining:
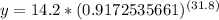

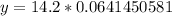
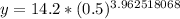
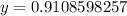
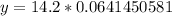
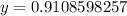
Since both the initial amount of Iodine, and the amount of time were given to 3 significant figures, the amount remaining after 31.8days is 0.911g.