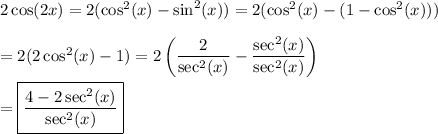Answer:
Explanation:
Several trig identities are involved in the proof of this. This is the order in which they are used.
- cos(2x) = cos²(x) -sin²(x)
- cos²(x) +sin²(x) = 1
- cos(x) = 1/sec(x)
Proof
Starting with the left side, we can transform it into the right side.