Answer:

❁ Question : Simplify :
❁ Solution :
First , Use power law of indices.
Remember : If
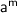 is an algebraic term , then
is an algebraic term , then
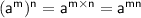 , where m and n are positive integers.
, where m and n are positive integers.
➝

➝
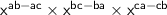
Now , Use product law of indices :
Remember : If
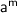 and
and
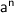 are the two algebraic terms , where m and n are the positive integers then
are the two algebraic terms , where m and n are the positive integers then
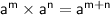
➝
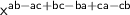
Since two opposites adds up to zero , remove them :
➝
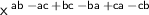
➝
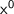
Use Law of zero index
Remember : If
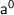 is an algebraic term , where a ≠ 0 , then
is an algebraic term , where a ≠ 0 , then
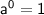
➝

And we're done !
Hope I helped ! ♡
♪ Have a wonderful day / night ツ
~~