Answer:
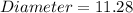 cm --- (a)
cm --- (a)
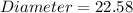 cm --- (c)
cm --- (c)
Explanation:
Only (a) & (c) are clear enough to be solved. Others are not properly presented.
Use the following explanations of (a) & (c) to answer others.
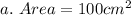
Area is calculated as:
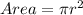
Substitute 100 for Area
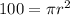
Take π as 3.14. So, the expression become

Divide both sides by 3.14
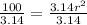
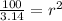
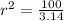
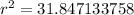
Solve for r
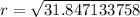
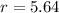
But diameter = 2 * radius
So:
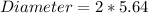
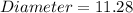

Area is calculated as:
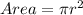
Substitute 400 for Area
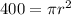
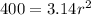
Divide both sides by 3.14
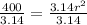
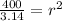
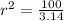

Solve for r

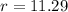
But diameter = 2 * radius
So:

