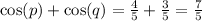Question

If sin Q= 4/5, cos P + cos Q = ____

Answer 1

We know that ,

`\sin( \alpha ) = (opposite)/(hypotenuse)`

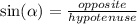
and

`\cos( \alpha ) = (adjacent)/(hypotenuse)`

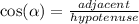
where 'alpha' is an angle of triangle ; 'opposite' denotes the side opposite to alpha & 'adjacent' refers to the side next to the angle (but not hypotenuse)

Similarly ,

`\sin(q) = (opposite)/(hypotenuse) = (4)/(5)`

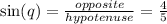
Let the length of the opposite side be 4x and the length of hypotenuse be 5x. By using Pythagorean Theorem , we can find the length of base.

`{base}^(2) + {(4x)}^(2) = {(5x)}^(2)`

`= > {base}^(2) = 25 {x}^(2) - 16 {x}^(2) = 9 {x}^(2)`

`= > base = \sqrt{9 {x}^(2) } = 3x`

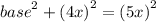
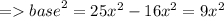
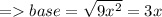
Now , we have got the length of all the sides of the triangle. So,

`\cos(q) = (adjacent)/(hypotenuse) = (3x)/(5x) = (3)/(5)`

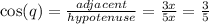
and

`\cos(p) = (adjacent)/(hypotenuse) = (4x)/(5x) = (4)/(5)`

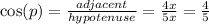
So,

`\cos(p) + \cos(q) = (4)/(5) + (3)/(5) = (7)/(5)`