Answer:
The difference is 117.3 cubic inches.
Explanation:
Radius of cylinder = 4 inches
Height of cylinder = 3 inches
Volume of a cylinder =

 h
h
Where r is the radius and h the height.
Volume of the given cylinder =
 x
x
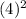 x 3
x 3
=
 x 16 x 3
x 16 x 3
= 150.857
Volume of the given cylinder = 150.86 cube inches
Radius of the sphere = 4 inches
Volume of sphere =


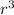
Volume of the given sphere =
 x
x
 x
x
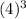
=
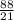 x 64
x 64
= 268.191
Volume of the sphere = 268.19 cube inches.
Difference between the volumes = 268.191 - 150.857
= 117.333
The difference is 117.3 cubic inches.