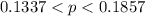Question

Environmental engineers are using data collected by weather data centers to learn how climate affects the sea ice. Of ice melt ponds studied in a certain​ region, were classified as having​ "first-year ice". The researchers estimated that about ​% of melt ponds in the region have​ first-year ice.​ Estimate, with​ 90% confidence, the percentage of all​ ice-melt ponds in the region that have​ first-year ice. Give a practical interpretation of the results.

Answer 1

Complete Question

Environmental engineers are using data collected by weather data centers to learn how climate affects the sea ice. Of 526 ice melt ponds studied in a certain​ region, 84 were classified as having​ "first-year ice". The researchers estimated that about 16% of melt ponds in the region have​ first-year ice.​ Estimate, with​ 90% confidence, the percentage of all​ ice-melt ponds in the region that have​ first-year ice. Give a practical interpretation of the results.

Answer:

The 90% confidence interval is
`0.1337   <  p < 0.1857`

Explanation:

From the question we are told that

The sample size is n = 526

The number that were classified to having a 'first-year ice ' is k = 84

The population proportion is p = 0.16

Generally the sample proportion is mathematically represented as

`\^ p = (k)/( n )`

=>
`\^ p = ( 84)/( 526 )`

=>
`\^ p = 0.1597`

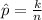
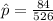
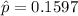
From the question we are told the confidence level is 90% , hence the level of significance is

`\alpha = (100 - 90 ) \%`

=>
`\alpha = 0.10`

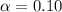
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  1.645`

Generally the margin of error is mathematically represented as

`E =  Z_{(\alpha )/(2) } * \sqrt{( p (1-  p))/(n) }`

=>
`E =  1.645 * \sqrt{(0.16 (1- 0.16))/( 526) }`

=>
`E =  0.026`

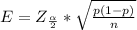
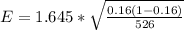
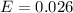
Generally 95% confidence interval is mathematically represented as

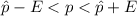
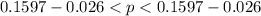
`\^ p -E <  p <  \^ p +E`

=>
`0.1597  -0.026  <  p < 0.1597  -0.026`

=>
`0.1337   <  p < 0.1857`