Question

Two cars are driving towards an intersection from perpendicular directions. The first car's velocity is 222 meters per second and the second car's velocity is 999 meters per second. At a certain instant, the first car is 888 meters from the intersection and the second car is 666 meters from the intersection. What is the rate of change of the distance between the cars at that instant (in meters per second)

Answer 1

`-7\ \text{m/s}`

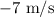
Step-by-step explanation:

x = Distance of 1st car = 8 m

y = Distance of second car = 6 m

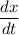`(dx)/(dt)` = Velocity of 1st car = 2 m/s

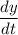`(dy)/(dt)` = Velocity of 2nd car = 9 m/s

Distance between the cars at the given instant

`r=√(x^2+y^2)\\\Rightarrow r=√(8^2+6^2)\\\Rightarrow r=10\ \text{m}`

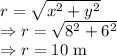
From pythagoras theorem we have

`r^2=x^2+y^2`

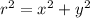
Differentiating with respect to time we get

`2r(dr)/(dt)=2x(dx)/(dt)+2y(dy)/(dt)\\\Rightarrow (dr)/(dt)=(x(dx)/(dt)+y(dy)/(dt))/(r)\\\Rightarrow (dr)/(dt)=(-8* 2-6* 9)/(10)\\\Rightarrow (dr)/(dt)=-7\ \text{m/s}`

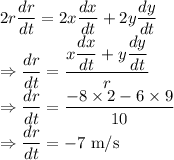
The rate of change of the distance between the cars at the given instant is
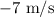`-7\ \text{m/s}`.