Question

A copper rod has a length of 1.7 m and a cross-sectional area of 3.2 × 10-4 m2. One end of the rod is in contact with boiling water and the other with a mixture of ice and water. What is the mass of ice per second that melts? Assume that no heat is lost through the side surface of the rod.

Answer 1

Given :

A copper rod has a length of 1.7 m and a cross-sectional area of
`3.2 * 10^(-4) \ m^2`.

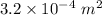One end of the rod is in contact with boiling water and the other with a mixture of ice and water.

To Find :

The mass of ice per second that melts.

Solution :

Amount of heat transfer from boiling water to ice through the copper bar is :

`Q = (kA\Delta T )/(L)\\\\Q = (390* 3.2 * 10^(-4) * 100)/(1.7)\\\\Q = 7.34 \ J/s`

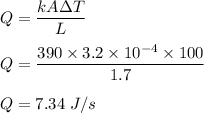
Let, amount of ice melts per second is :

`m=(Q)/(L_f)`

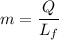
Here,
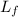`L_f` is the latent of fusion of water and is equal to
`L_f = 3.34* 10^5 \ J/s`.

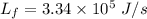Putting all values in above equation, we get :

`m=(7.34)/(3.34* 10^5)\\\\m = 2.19 * 10^(-5) \ kg/s`

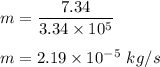
Hence, this is the required solution.