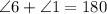Answer:
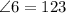
Explanation:
Given
Lines x and y
Transversal b
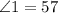
Required
Find

From the attachment,
 and
and
 are vertically opposite.
are vertically opposite.
This means that

Similarly
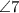 and
and
 are supplementary angles
are supplementary angles
So:
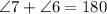
Make
 the subject
the subject
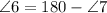
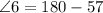
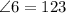
The relationship between
 and
and
 is that they are supplementary angles because:
is that they are supplementary angles because: