Question

What I the range of f(x)=4^x?

A. all positive real numbers
B. all real numbers
C. all real numbers grater that or equal to 4
D. all real numbers grater than 4

Answer 1

We conclude that the range of the function is 'all positive real numbers'.

Thus, option (A) is true.

Explanation:

Given the expression

`f\left(x\right)=4^x`

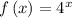
Determining the domain:

The domain of a function is the set of input values for which the function is real and defined.

It is clear that the given function has no undefined points nor domain constraints.

Therefore, the domain is: -∞ < x < ∞

Thus,

`\mathrm{Domain\:of\:}\:4^x\::\quad \begin{bmatrix}\mathrm{Solution:}\:&\:-\infty \:<x<\infty \\ \:\mathrm{Interval\:Notation:}&\:\left(-\infty \:,\:\infty \:\right)\end{bmatrix}`

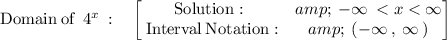
Determining the range:

The range is the set of values of the dependent variable for which a function is defined.

We know that the range of an exponential function of the form

`c\cdot \:n^(ax+b)+k\:\mathrm{is}\:\:f\left(x\right)>k`

`k=0`

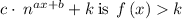
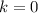
In other words, the range is all positive real numbers.

Thus,

`\mathrm{Range\:of\:}4^x:\quad \begin{bmatrix}\mathrm{Solution:}\:&\:f\left(x\right)>0\:\\ \:\mathrm{Interval\:Notation:}&\:\left(0,\:\infty \:\right)\end{bmatrix}`

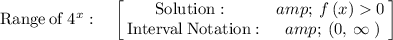
Therefore, we conclude that the range of the function is 'all positive real numbers'.

Thus, option (A) is true.