Answer:
Max Value: x = 400
General Formulas and Concepts:
Algebra I
- Domain is the set of x-values that can be inputted into function f(x)
Calculus
- Antiderivatives
- Integral Property:
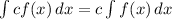
- Integration Method: U-Substitution
- [Integration] Reverse Power Rule:
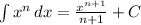
Explanation:
Step 1: Define
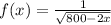
Step 2: Identify Variables
Using U-Substitution, we set variables in order to integrate.
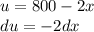
Step 3: Integrate
- Define:
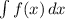
- Substitute:

- [Integral] Int Property:
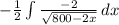
- [Integral] U-Sub:
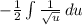
- [Integral] Rewrite:
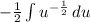
- [Integral - Evaluate] Reverse Power Rule:
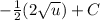
- Simplify:
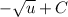
- Back-Substitute:

- Factor:

Step 4: Identify Domain
We know from a real number line that we cannot have imaginary numbers. Therefore, we cannot have any negatives under the square root.
Our domain for our integrated function would then have to be (-∞, 400]. Anything past 400 would give us an imaginary number.