Question

Determine whether LINE AB and LINE CD are parallel, perpendicular, or neither. A(−1, −4) , B(2, 11) , C(1, 1) , D(4, 10) ( write parallel, perpendicular, or neither

Answer 1

Explanation:

Hey there!

The points of line AB are; (-1,-4) and (2,11).

Note:

• Use double point formula and simplify it to get two eqaution.
• Use condition of parallel lines, perpendicular lines to know whether the lines are parallel or perpendicular or nothing.

~ Use double point formula.

`(y - y1) = (y2 - y1)/(x2 - x1) (x - x1)`

~ Keep all values.

`(y + 4) = (11 + 4)/(2 + 1) (x + 1)`

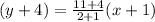
~ Simplify it.

`y + 4 = (15)/(3) (x + 1)`

`y + 4 = 5x + 5`

`5x - y + 1 = 0`

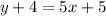
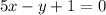
Therefore this is the equation of line AB.

Now, Finding the equation of line CD.

Given;

The points of line CD are; (1,1) and (4,10).

~ Using formula.

`(y - y1) = (y2 - y1)/(x2 - x1)(x - x1)`

~ Keep all values.

`(y - 1) = (10 - 1)/(4 - 1) (x - 1)`

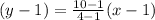
~ Simplify it.

`y - 1 = 3 x - 3`

`3x - y - 2 = 0`

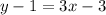
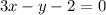
Therefore, 3x - y- 2 = 0 is the eqaution of line CD.

Use condition of parallel lines.

m1= m2

Slope of equation (i)

`m1 = ( - coeff. \: of \: x)/(coeff \: of \: y)`

`m1 = ( - 5)/( - 1)`

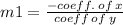
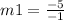
Therefore, m1 = 5

Slope of second equation.

`m2 = ( - coeff \: .of \: x)/(coeff \: .of \: y)`

`m2 = ( - 3)/( - 1)`

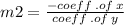
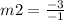
Therefore, m2 = 3.

Now, m1≠m2.

So, the lies are not parallel.

Check for perpendicular.

m1*m2= -1

3*5≠-1.

Therefore, they aren't perpendicular too.

So, they are neither.

Hope it helps...