Question

What is the x-coordinate of the point that divides the directed line segment from J to K into a ratio of 2:5? X = (StartFraction m Over m + n EndFraction) (x 2 minus x 1) + x 1 –4 –2 2 4

Answer 1

B) -2

Explanation:

Answer 2

-2

Explanation:

The equation of a segment C(x, y) that divides the line segment AB with endpoints A(
`x_1,y_1`) and B(
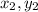`x_2,y_2`) in the ratio m:n is:

`x=(m)/(n+m) (x_2-x_1)+x_1\\\\y=(m )/(n+m) (y_2-y_1)+y_1`

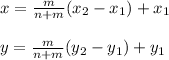
A point (x, y) divides the directed line segment from J(-6, -2) to K(8, -9) into a ratio of 2:5. Hence:

`x=(2)/(2+5)(8-(-6))+(-6)\\\\x=4-6\\\\x=-2\\\\`

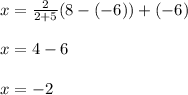
Also:

`y=(2)/(2+5)(-9-(-2))+(-2)\\\\y=-2-2\\\\y=-4`

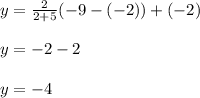
The point is (-2, -4)