Question

Do the following pairs of equations represent parallel lines,

perpendicular lines, or neither; y = -1/2 x + 5 and y = -1/2 x - 9?

Answer 1

Parallel

Explanation:

What makes two lines parallel?

For two lines to be parallel, they must have the same slope.

These are linear equations, which are represented by
`y=mx+b` where m is the slope and b is the y-intercept.

In this pair of equations, we can see that the m (or the slope) are exactly the same (
`-(1)/(2) = -(1)/(2)`). This means that they are parallel.

What makes two lines perpendicular?

Two lines are perpendicular when the slope of one of them is equal to the negative reciprocal of the other.

For example, to find the perpendicular line to
`y=-(1)/(2)x+5` we would get the m (
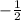`-(1)/(2)`) and find its negative reciprocal.

`-(-(2)/(1)) = 2`

We can verify this graphically by using a calculator (if you have a graphing calculator) or we can use the Desmos tool (online graphing calculator). Please see the image that's attached to this answer. It's evident that they are perpendicular in this image due to the fact that they make an angle of 90 degrees.

Let me know if there's something you'd like me to explain further. Good luck!