Question

Find the zeros of the polynomial
function and state the multiplicity of
each

Answer 1

Hi the answer zero ther u go

Answer 2

The zeros of the polynomial function
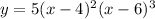`y=5(x-4) ^2 (x-6) ^3`and their multiplicities are:

4 with multiplicity 2

6 with multiplicity 3

The multiplicity of a zero is the number of times it appears in the factored form of the polynomial. In this case, the factor (x−4) appears twice, so the zero at x=4 has multiplicity 2. The factor (x−6) appears three times, so the zero at x=6 has multiplicity 3.

To find the zeros of a polynomial, we can set the polynomial equal to zero and solve the resulting equation. In this case, we have:

`5(x-4)^2(x-6)^3 = 0`

This equation is true if any of the factors is equal to zero. So, we have three equations to solve:

x-4 = 0

x-6 = 0

x-6 = 0

The first equation has the solution x=4. The second equation has the solution x=6. The third equation has the solution x=6.

Therefore, the zeros of the polynomial are x=4 and x=6. The zero at x=4 has multiplicity 2 and the zero at x=6 has multiplicity 3.