Answer:
Explanation:
Question 1.
Slope of line 'l' =
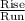
=

=

Equation of a line passing through (x', y') and slope 'm' is,
y - y' = m(x - x')
Since, line 'l' is passing through (0, 7) and slope =

Equation will be,
y - 7 =

y =
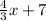
Similarly, slope of line m =
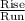
=
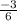
=
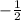
Therefore, equation of line 'm' passing through point (0, -4) will be,
y + 4 =

y =
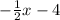
Solution = Point of intersection of both the lines
= (-6, -1)
Question 2
Slope of line 'l' =
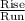
=
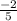
Equation of line 'l' passing through a point (0, 4) and slope = -

y - 4 =
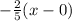
y =

Slope of line 'm' =
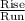
=
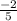
Equation of line 'm' passing through a point (0, -1) and slope =
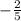
y + 1 =
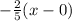
y =
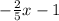
Since, slopes of both the lines are same, these lines will be parallel.
There will be NO SOLUTION.