Answer:
The slope is 0.55
Explanation:
Right Triangles
The string, the ground, and the height of the balloon form a right triangle where the trigonometric ratios are satisfied.
The image below shows the geometric construction. We are required to find the slope the string forms with the ground.
It can be found by using the tangent ratio:
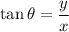
Substituting y=30 and x=55
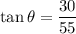
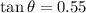
The slope is 0.55