Question

1) Using the equation find a, b, x1 and y1​

Answer 1

• (x₁, y₁) = (2, 1)

• a = 4, b = 2

Explanation:

The standard form equation of a hyperbola is

`(\left(x-h\right)^2)/(a^2)-(\left(y-k\right)^2)/(b^2)=1`

Here:

• center (x₁, y₁) = (h, k)

• 'a' is semi-axis and 'b' is semi-conjugate axis 'b'

Given the equation

`(\left(x-2\right)^2)/(4^2)-(\left(y-1\right)^2)/(2^2)=1`

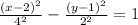
comparing with the standard form equation of a hyperbola

(x₁, y₁) = (h, k) = (2, 1)

a = 4, b = 2

Therefore,

• (x₁, y₁) = (2, 1)

• a = 4, b = 2