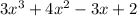Question

The Johnsons’ new swimming pool measures 3x^2-2x+1 ft in length and x+2 ft in width. What is the area of the new swimming pool, in square feet?

Answer 1

`3x^3 + 4x^2 -3x + 2`

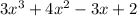
Explanation:

The area of the swimming pool will be equal to
`length * width`.

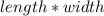We know that the length is
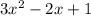`3x^2-2x+1` and the width is
`x+2`.

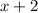Therefore we can substitute those into the equation:

`(3x^2-2x+1)(x+2)\\`

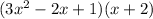
Then, we expand the brackets using a grid (attached).

After this, we collect like terms to find the final answer:

`3x^3+6x^2-2x^2-4x+x+2\\3x^3 + 4x^2 -3x + 2`

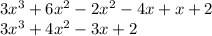
Our final answer is:
`3x^3 + 4x^2 -3x + 2`