Question

(3x^2 + 2x - 3)(x - 1)

Answer 1

`3x^3-x^2-5x+3`

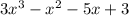
Explanation:

This is purely a case of expanding brackets.

Let's mark down our original equation:

`(3x^2 +2x-3)(x-1)`

It may be helpful the draw a grid to help you expand this equation (attached).

You construct these diagrams by taking the
`ax^2`,
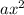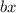`bx` and
`c` elements of one bracket and placing them in headers, and do the same for the other bracket, but in the left hand side of the table. Then, like a multiplication grid, you multiply all the values across. At the end, you collect all your like terms:

`3x^3 -3x^2+2x^2-2x-3x+3\\3x^3-x^2-5x+3`

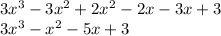
So, our final answer is
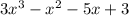`3x^3-x^2-5x+3`.

Answer 2

3x^3 - x^2 - 5x + 3

Distributive property of multiplication
×(3x^2 + 2x - 3) - 1(3x^2 + 2x - 3)
3×^3 + 2x^2-3x-3x^2-2×+3
Group like terms:
3x^3+2×^2-3x^2-3x-2×+3
3x^3-x^2-5x+3